ABSTRACT
Because reasoning about mechanism is critical to disciplined inquiry in science, technology, engineering, and mathematics (STEM) domains, this study focuses on ways to support the development of this form of reasoning. This study attends to how mechanistic reasoning is constituted through mathematical description. This study draws upon Smith’s (2007) characterization of mathematical description of scientific phenomena as ‘‘bootstrapping,’’ where negotiating the relationship between target phenomena and represented relations is fundamental to learning.
In addition, the development of mathematical representation presents a viable pathway towards STEM integration. In this study, participants responded to an assessment of mechanistic reasoning while cognitive interviews were conducted to characterize their reasoning about mechanism and mathematical description of the systems of levers represented in the items. Participant item responses were modeled using item response theory and participant talk and gesture were coded according to developed analytic frameworks.
Participants were elementary, middle, and high school students as well as college undergraduates, and adults without college education. The results suggest a relationship between participants’ tendencies to describe these systems mathematically and their mechanistic reasoning ability. Moreover, there are specific elements of mechanistic reasoning that are more highly associated with mathematical description. In addition, there is a relationship between a participant’s propensity to both mathematically describe and mechanistically trace mechanical systems.
STEM INTEGRATION AND MATHEMATICAL DESCRIPTION
One challenge facing science, technology, engineering, and mathematics (STEM) education is integration, advancing student conceptual development within and across STEM domains (National Research Council [NRC], 2007). The National Academies Press (NAE) (Honey, Pearson, & Schweingruber, 2014) has argued that integrated STEM education should bring together concepts from more than one discipline (e.g., mathematics and science, or science, technology, and engineering); it may connect a concept from one domain to a practice of another, such as applying properties of geometric shapes (mathematics) to engineering design (Weinberg, 2012); or it may combine two practices, such as scientific inquiry (which include modeling, argument, etc.) and engineering design (in which data from a science experiment can be applied).
MATHEMATICAL DESCRIPTION AND CONCEPTUAL CHANGE
This study highlights that the difficulty with mechanistic reasoning emerges from the contexts in which participants are asked to employ conceptual resources and not from the absence or presence of said resources. For instance, being able to perceive and diagnose the motion of a lever in one context does not imply the propensity to immediately perceive and diagnose all other levers’ motions in all contexts where they appear. Disciplining one’s perception to ‘‘see’’ in such a way as to diagnose lever motion across lever types and arrangements no doubt takes time and experience within a legitimate situated context (Lave & Wenger, 1991; Stevens & Hall, 1998) (e.g., K–12 STEM education).
MECHANISTIC REASONING AND MATHEMATICAL DESCRIPTION
Kobiela et al. (2011) have shown that when students engage in the mathematical description of systems of levers it makes their mechanisms more salient and comprehensible. Their study characterizes the relationship between mathematical description and mechanistic reasoning about systems of levers within engineering design. In addition, it investigates how mathematical description mediates the mechanistic tracing of these systems from input to output.
RESEARCH QUESTIONS
This study characterizes the ways that participants spontaneously deploy mathematical description to buttress mechanistic reasoning. The following research questions are addressed: (1) in what ways do participants mathematically describe simple systems of levers and (2) how does this mathematical description mediate their mechanistic reasoning about these systems?
METHOD
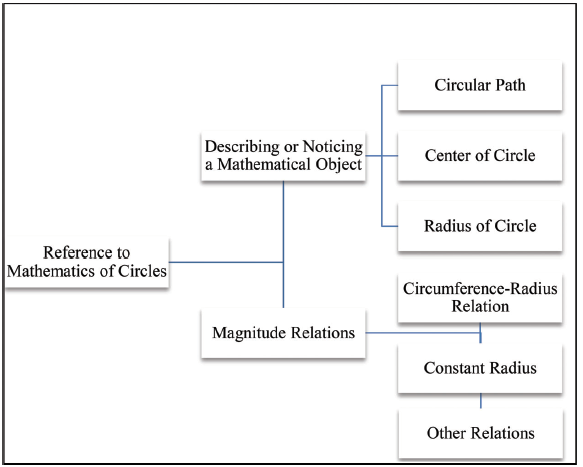
Figure 1. Framework for mathematical description.
Mathematical description was categorized according to the following: (1) describing or noticing a mathematical object and (2) attending to magnitude relations (Figure 1). Describing or noticing a mathematical object included the following subcategories: (a) circular path (‘‘this lever goes in a circle’’) (Constance, grade 3), (b) center of circle (‘‘this lever moves around this point,’’) (Kim, undergraduate/ engineering major), and (c) radius of circle (‘‘the part of the link that will move up is from here [fulcrum] to here [end of lever]) (Roderick, grade 9; underline highlights mathematical description).
RESULTS
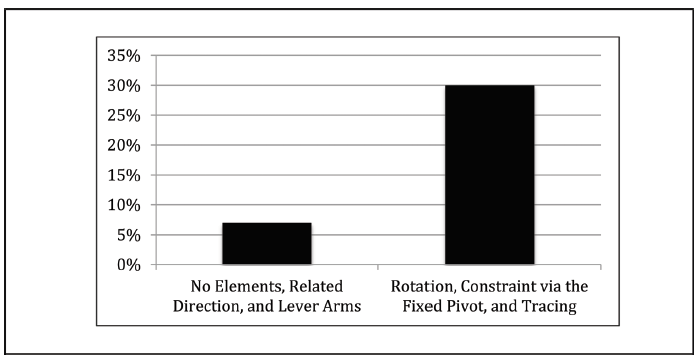
Figure 3. Percentage of relational mathematical description by groups of mechanistic elements.
Those participants who showed the proclivity to mathematically describe the levered system were all coded at the level of describing or noticing mathematical objects. However, those participants who were diagnosed at the level of rotation, constraint via the fixed pivot, and tracing cited magnitude relations more than those who did not (p < 0.0001; Chi-squared test; Figure 3).
An example of tracing while mathematically describing the mechanistic elements, from input to output, on an assessment item is presented in Figure 4. Kelly, an undergraduate engineering major, provided evidence of tracing and mathematical description on this paper-and-pencil representation of a system of levers.
DISCUSSION
This study focuses on the development of mechanistic reasoning in science and engineering education and how such reasoning is constituted through mathematical description. Because these forms of reasoning are context specific (diSessa, 1993), this study focused on simple systems of levers. Even though children have conceptual resources for these forms of reasoning under specific conditions, they do not necessarily deploy these forms of reasoning spontaneously in unfamiliar or untutored contexts.
This may be the case because these forms of reasoning are heavily dependent on the cues that are provided (and attended to) when participants are considering which components are relevant to system functioning. Many characteristics of simple machines, including aspects of their appearance, embeddedness within other components, and possibly other attributes as well, will likely influence the difficulty with which individuals develop and support mechanistic explanations from a system’s input to output. Smith (2007) describes ‘‘bootstrapping’’ as a means to facilitate this context-specific reasoning, whereby individuals negotiate the relationships between systems of levers and their represented relations.
For instance, participants that spontaneously referenced mathematics to explain machine motion, on at least one item, had higher mechanistic reasoning ability scores on the assessment (M = 0.43 logits; p < 0.0001, one-tailed t-test) than those who did not reference mathematics in their explanations (M = -1.38). Moreover, with each additional reference to mathematics there was an increase in assessed mechanistic reasoning ability. Thus, the capacity to seek out mechanism seems to be constituted through the development of represented resources. This work suggests an available entree into STEM integration because the development of mechanistic reasoning (i.e., in science and engineering) relies upon representational resources from mathematics.
Source: Oakland University
Authors: Paul J. Weinberg
